MACHINE SHOP CALCULATION - OD1640 - LESSON 1/TASK 1
EXAMPLE
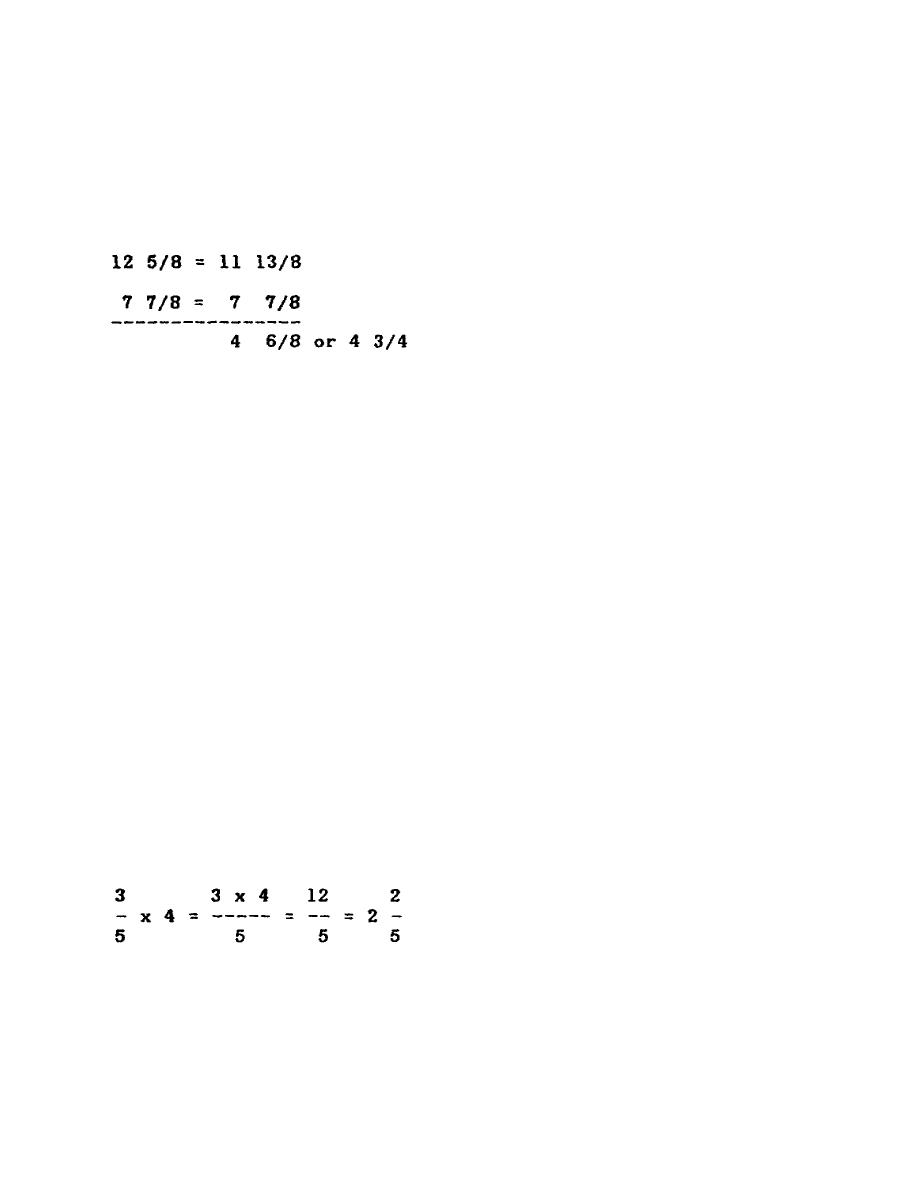
From 12 5/8 take 7 7/8. 7/8 cannot be subtracted from 5/8, so borrow 8/8,
or 1, from the 12; add this 8/8 to the 5/8, thus:
8.
a.
Finding the Product of a Fraction.
In multiplying fractions, they
need not be reduced to an LCD, as in adding and subtracting fractions. When
the numerator of a fraction is multiplied, the number of fractional units is
multiplied. Their size (represented by the denominator) remains the same.
But to multiply or increase the size of the fractional units (represented by
the denominator), the denominator must be divided, and the number of
fractional units (represented by the numerator) remains the same.
Before
multiplying, it is recommended that canceling of equal factors be carried
out.
(Cancellation is the process of striking out equal factors from the
numerator and denominator of a fraction. This operation does not change the
value of the fraction but aids in reducing it to its lowest terms.)
Rule 1.
To multiply a fraction by an integer (a whole number), or an
integer by a fraction, multiply the numerator by the integer.
Reduce the
product to its lowest terms. To multiply a fraction by a fraction, multiply
the numerators together.
This gives the numerator of the product.
Next,
multiply the denominators together.
This gives the denominator of the
product. Cancel where possible.
EXAMPLE
Multiply 3/5 by 4.
This means find a fraction 4 times as great as 3/5.
17



 Previous Page
Previous Page
